Ryan P. A. BETTENS

Associate Professor

Research Fellow, RSC, Australian National University, 1998-2000; Postdoc. Fellow, RSC, Australian National University, 1996-1998; Postdoc. Research, Ohio State University, 1994-1996; Postdoc. Research, ETH, Zurich, 1992-1994; Ph.D., Monash University, 1992; B.Sc., University of Queensland, 1986.

Contact Information:

Office: MD1-05-03C

Tel: (65)-6516-2846

Fax: (65)-6779-1691

Email: chmbrpa@nus.edu.sg

Research

ORCID: 0000-0003-1285-1817

ResearcherID: I-1549-2015

Research Interests

My research area is in the understanding and accurate description, via computational chemistry, of inter- and intra-molecular interactions. Ultimately the understanding and description will be applied to the dynamics of large systems like proteins, nucleic acids and bulk matter. Specific foci are:

Accurately describe and predict small molecule potential energy surfaces.
Accurately describe water and its interactions with large molecules.
Machine learning applied to the prediction of potential energy surfaces and other areas of chemistry.

Research Highlight

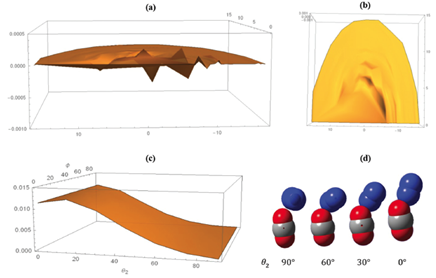
Ref: Wang, H.; Bettens, R. P. A., Modelling Potential Energy Surfaces for Small Clusters Using Shepard Interpolation with Gaussian-Form Nodal Functions. Phys. Chem. Chem. Phys. 2019, 21, 4513-4522. In the highlighted paper we examined a novel approach to accurately predicting the potential energy surface (PES) of a chemical system when a nuclear configuration is given as input. The PESs of small atmospheric clusters have theoretical as well as environmental significance. A common method used to generate analytical PESs is the Shepard interpolation, where the PES is a weighed sum of Taylor series expansions (nodal functions) at ab initio sample points. Based on this, we presented a new method based on the Shepard interpolation, where the nodal functions are composed of a symmetric Gaussian term and an asymmetric exponential term in each dimension. Corresponding sampling methods were also developed. We tested the method on several atmospheric bimolecular clusters and achieved root mean square errors (RMSE) below 0.13 kJ mol^-1 in 150 samples for Ar–rigid H₂O and Ne–rigid CO₂, and below 0.39 kJ mol^-1 in 1800 samples for rigid N2–rigid CO₂.

Below: Error of the estimated PES of CO₂–N₂. (a) Plot of error on a 200-point test set against R and θ₁ of the test points. Energy in E_h. Box dimensions in a₀. (b) The top view of the error plot; (c) ab initio energy of configurations with R = 7.25a₀, θ₁ = 30°. The energy is sensitive to θ₁ much more than to ∅. (d) Space-filling models of configurations with R = 7.25a₀, θ₁ = 30°, ∅=60° and various θ₂ using van der Waals radii. Both colliding and non-colliding configurations are present.

Teaching Contributions AY2022/2023

CM2133 Foundations of Physical Chemistry

Representative Publications

Wang, H.; Bettens, R. P. A., Modelling Potential Energy Surfaces for Small Clusters Using Shepard Interpolation with Gaussian-Form Nodal Functions. Phys. Chem. Chem. Phys. 2019, 21, 4513-4522.
Ng, Y.-H.; Bettens, R. P. A., Improving on Equilibrium Isotropic Nuclear Shielding Constants. Inter. J. Quant. Chem. 2017, 117, 15-23.
Ng, Y.-H.; Bettens, R. P. A., Comparing Vibrationally Averaged Nuclear Shielding Constants by Quantum Diffusion Monte Carlo and Second-Order Perturbation Theory. J. Phy. Chem. A. 2016, 120, 1297-1306.
Ouyang, J. F.; Bettens, R. P. A., When are Many-Body Effects Significant? J. Chem. Theory Comput. 2016, 12, 5860-5867.
Ouyang, J. F.; Bettens, R. P. A., Many-Body Basis Set Superposition Effect. J. Chem. Theory Comput. 2015, 11, 5132-5143.
Collins, M. A.; Bettens, R. P. A., Energy-Based Molecular Fragmentation Methods. Chem. Rev. 2015, 115, 5607-5642.
Collins, M. A.; Cvitkovic, M. W.; Bettens, R. P. A. The Combined Fragmentation and Systematic Molecular Fragmentation Methods. Acc. Chem. Res. 2014, 47, 2776-2785.
Ouyang, J. F.; Cvitkovic, M. W.; Bettens, R. P. A., Trouble with the Many-Body Expansion. J. Chem. Theory Comput. 2014, 10, 3699-3707.